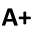1. Red Dragon is concerned that one or more of the new delivery people might be slower than the others.
(a) Find 95% confidence intervals for the mean travel time and the mean total time for each delivery person (the total time is the sum of the travel and prep times). Are these fair measures for comparing the efficiency of the delivery people?
(b) Find a 95% confidence interval for the mean speed of delivery for each delivery person, where speed is measured as miles per hour during the trip from the restaurant to the customer.
2. Red Dragon would like to advertise that it can achieve a total delivery time of no more than M minutes for all customers within a 10-mile radius. On all orders that take more than M minutes, Red Dragon will offer the customers a £10 certificate on their next purchase.
(a) Calculate the proportion of deliveries (within the 10-mile limit) that will be on time if M = 25, 30, or 35 minutes.
(b) Let us call the proportion of on-time deliveries p. There is a known expression for the confidence interval of this quantity:
![]()
where n is the sample size.
Assume for now that the delivery people in the sample are representative of all Red Dragon's delivery people. Find a 95% confidence interval for the proportion of deliveries (within the 10-mile limit) that will be on time if M = 25, 30, or 35 minutes. NOTE: The square root function in Excel is SQRT.
(c) Suppose that Red Dragon makes 1000 deliveries within the 10-mile limit. For each of the values of M in part (a) above, find a 95% confidence interval for the total £ amount of certificates it will have to give out for being late.
3. Test whether there is a significant difference between the mean speeds for each pair of delivery people.
Question 2
Read the Store 24 case (on Moodle under Session 5) and answer the following questions.
. i) What question does Paul Doucette want to use data to answer? How would you suggest Sarah to answer this question?
. ii) Beyond the above question Paul Doucette is interested, what is Bob Gordon interested in using the data to answer? How can Sarah answer Bob’s questionusing data?
. iii) Tom Hart has a lot of experience in the field. What is his observation regarding store performance and tenure? How can Sarah test that using data?